What Is Commutative Property Of Addition?

Commutative Property Of Addition: There are three basic properties of numbers, and your textbook will probably have just a little section on these properties, somewhere near the beginning of the course, and then you’ll probably never see them again (until the beginning of the next course). My impression is that covering these properties is a holdover from the “New Math” fiasco of the 1960s. While the topic will start to become relevant in matrix algebra and calculus (and become amazingly important in advanced math, a couple of years after calculus), they really don’t matter a whole lot now.

Why not? Because every math system you’ve ever worked with has obeyed these properties! You have never dealt with a system where a×b did not, in fact, equal b×a, for instance, or where (a×b)×c did not equal a×(b×c). This is why the properties probably seem somewhat pointless to you. Don’t worry about their “relevance” for now; just make sure you can keep the properties straight so you can pass the next test. The lesson below explains how I keep track of the properties.

What Is Commutative Property Of Addition?

What is associative and commutative property of addition?

Commutative Properties of Multiplication

The associative property states that you can re-group numbers and you will get the same answer and the commutative property states that you can move numbers around and still arrive at the same answer.

What are the 4 properties of addition?

There are four mathematical properties that involve addition. The properties are the commutative, associative, identity, and distributive properties. Commutative Property: When two numbers are added, the sum is the same regardless of the order of the addends.

Commutative Property Of Addition Example

The commutative property of addition says that changing the order of addends does not change the sum. Here’s an example:

4, plus, 2, equals, 2, plus, 4

Notice how both sums are 6 even though the ordering is reversed.

[Help me understand how this works!]

Here’s another example with more addends:

Commutative Property Of Addition Definition

We begin with the definition of the commutative property of addition. Simply put, it says that the numbers can be added in any order, and you will still get the same answer. For example, if you are adding one and two together, the commutative property of addition says that you will get the same answer whether you are adding 1 + 2 or 2 + 1.

This also works for more than two numbers. Say you are adding one, two and three together (1 + 2 + 3). The commutative property of addition says that you can also add 2 + 1 + 3 or 3 + 2 + 1 and still get the same answer.

Let’s think about marbles for a minute. Let’s say we have two groups of marbles. One group only has one marble and the other group has two marbles. How many marbles do we have all together? We have three. Now, does it matter where you place your groups of marbles? For example, how many marbles will you have if you have one marble at the top of the stairs and two marbles at the bottom of the stairs? We still have three; we just have to climb the stairs to get all the marbles.

Now, what if you switched the two groups so that you have two marbles at the top of the stairs and one marble at the bottom of the stairs? How many total marbles will you have? You still have three. It doesn’t matter where you place your groups of items, you will still have the same total. This is what the commutative property of addition is all about.

What Is The Commutative Property Of Addition

The Distributive Property is easy to remember if you recall that “multiplication distributes over addition”. Formally, they write this property as “a(b + c) = ab + ac“. In numbers, this means, for example, that 2(3 + 4) = 2×3 + 2×4. Any time they refer in a problem to using the Distributive Property, they want you to take something through the parentheses (or factor something out); any time a computation depends on multiplying through parentheses (or factoring something out), they want you to say that the computation used the Distributive Property.

Why is the following true? 2(x + y) = 2x + 2y

Since they distributed through the parentheses, this is true by the Distributive Property.

Use the Distributive Property to rearrange: 4x – 8

The Distributive Property either takes something through parentheses or else factors something out. Since there aren’t any parentheses to go into, you just need to factor out of. Then the answer is:

By the Distributive Property, 4x – 8 = 4(x – 2).

“But wait!” I hear you cry; “the Distributive Property says multiplication distributes over addition, not over subtraction! What gives?” You make a good point. This is one of those times when it’s best to be flexible. You can either view the contents of the parentheses as the subtraction of a positive number (“x – 2“) or else as the addition of a negative number (“x + (–2)“). In the latter case, it’s easy to see that the Distributive Property applies, because you’re still adding; you’re just adding a negative.

The other two properties come in two versions each: one for addition and the other for multiplication. (Yes, the Distributive Property refers to both addition and multiplication, too, but it refers to both of the operations within just the one rule.)

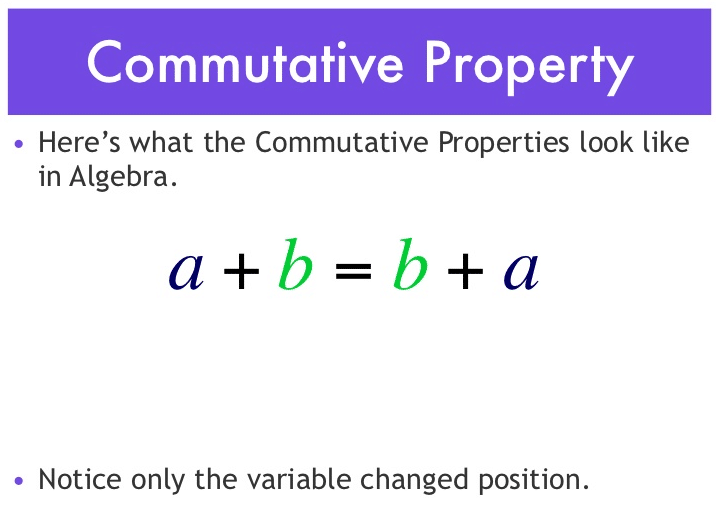
The Commutative Property Of Addition

The word “associative” comes from “associate” or “group”; the Associative Property is the rule that refers to grouping. For addition, the rule is “a + (b + c) = (a + b) + c“; in numbers, this means 2 + (3 + 4) = (2 + 3) + 4. For multiplication, the rule is “a(bc) = (ab)c“; in numbers, this means 2(3×4) = (2×3)4. Any time they refer to the Associative Property, they want you to regroup things; any time a computation depends on things being regrouped, they want you to say that the computation uses the Associative Property.

Rearrange, using the Associative Property: 2(3x)

They want me to regroup things, not simplify things. In other words, they do not want me to say “6x“. They want to see me do the following regrouping:

(2×3)x