Currently Empty: $0.00
Let’s Understand More About Volume Of a Prism
The volume of a prism is the total area inhabited by a prism. In this short article, you will certainly discover just how to find a prism volume by using the Volume of a prism formula.
Before we get started, let’s very first review what a prism is. By definition, a prism is a solid geometric number with two similar ends, level faces, and also the same cross-section, all along with its Size.
Prisms are called after the forms of their cross-section. For instance, a prism with a triangular cross-section is referred to as a triangular prism. Other instances of prisms consist of a rectangular prism. pentagonal prism, hexagonal prism, trapezoidal prism and so on
Identify Volume of a Prism
To discover the volume of a prism, you need the location and height of a prism. The Volume of a prism is determined by increasing the base location and the elevation. The Volume of a prism is also gauged in cubic units, i.e., cubic meters, cubic centimetres, etc.
The volume of a Prism
Solution
The formula for determining the Volume of a prism relies on the cross-section or Base of a prism. Because we already know the formula for computing the polygons’ location, locating the Volume of a prism is as easy as pie.
The basic formula for the volume of a prism is;
The Volume of a Prism = Base Area × Size
Where Base in the shape of a polygon is extrude to form a prism
Let’s review the Vol. of various kinds of prisms.
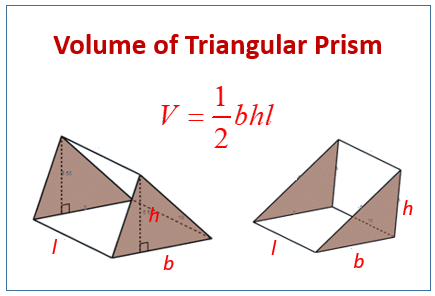
Vol. of a triangular prism.
It is a prism whose cross-section is a triangle, and the formula to find the vol. of a triangular prism is;
The vol. of a triangular prism = 1/2 ABH
where,
a = apothem of a triangular prism.
The polygon’s apothem is the line linking the polygon facility to one of the polygon’s sides’ navel. Also, the apothem of a triangle is the height of a triangular
b = base size of a triangle.
h = height of a prism.
Example
Locate the Vol. of a triangular prism whose apothem is 12 centimetres.
The base length is 16 centimetres and elevation is 25 cm.
Solution
By the formula of a triangular prism,
Volume = 1/2 abh
= 1/2 x 12 x 16 x 25
= 150 cm3
Vol. of a pentagonal prism
For a pentagonal prism, the Vol. is:
Let’s take a look
Vol. of a pentagonal prism = (5/2) abh
Where,
a = apothem of a government
b = base length of a pentagonal prism
h = elevation of a prism.
Example
Find the vol. of a pentagonal prism whose apothem is 10 cm, the base size is 20 cm and height, is 16 cm.
Solution
Vol. of a pentagonal prism = (5/2) abh
= (5/2) x 10 x 20 x 16
= 8000 cm3
The vol. of a hexagonal prism
A hexagonal prism has a hexagon as the cross-section or Base. The Vol. of a hexagonal prism is:
The vol. of a hexagonal prism = 3abh
where,
a = apothem length of a hexagon
b = base size of a hexagonal prism
h = elevation of a prism.
Example
Determine the vol. hexagonal prism with the apothem as 5 m, base length as 12 m, and elevation as 6 m.
Solution
Hexagonal prism = 3abh
= 3 x 5 x 12 x 6
= 1080 m3.