How to Find the Y Intercept of a Linear Equation
If you are looking for a way to find the y intercept of a linear equation, then you have come to the right place. This article will show you how to use a simple formula to determine the y-intercept of any straight line. You will also learn how to find the y-intercept of a quadratic equation using a graph and table.
Method to Calculate a straight line’s y-intercept
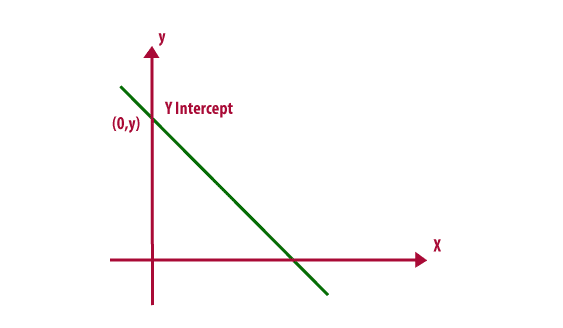
The y-intercept is the point on a graph where a straight line crosses a y-axis. The y-intercept is the same as the x-intercept, but in a different place. It can be found by figuring out the equation of a straight line.
To determine the y-intercept, you must first find the value of y for x=0. This is done by dividing the x-coordinates. X is the axis that the line is on. If you know the value of x, you can substitute it into the equation. You may want to use a calculator to help you with this process. A good calculator can provide you with an answer within seconds.
Another method of finding y-intercepts is to use the slope-intercept form of an equation. This type of equation has a constant y-intercept. This equation can be plotted as follows: y = mx + c, where m is the slope and c is the y-intercept. In this equation, c can be either positive or negative. Alternatively, you can also write y = mx+b.
Read Also: What Is Commutative Property Of Addition?
When using the slope-intercept form, you can use a and b to explain the position of the line. For example, when you are calculating the population in the United States, you can use y=0 to represent the size of the population in a year at the start of the population change. Similarly, when you are calculating a change in population, you can use y=0 to compare the population at the beginning of the change and the population at the end of the change.
For example, if a vertical line has a -6 slope, the line will have a y-value of -3. A vertical line with a -5 slope will have a y-value of 4 and a line with a -0.5 slope will have a y-value -2. Vertical lines have lots of “rise” in their x-coordinates, which means they are usually steeper than horizontal lines. But when a line has no “rise”, the line’s y-values are not going to be very steep.
Finding the y-intercept of a quadratic equation
The y-intercept of a quadratic equation is the point on the graph where the parabola of the function crosses the y axis. A quadratic equation is a mathematical function that can be written as y = ax2+bx+c. Its x coordinates are always 0. In addition, the y-intercept has a corresponding value that is equal to the c constant. This value can be factored and then used to solve a quadratic equation for a specific x value. There are several ways to find the y-intercept of a quadratic formula.
One of the easiest ways to find the y-intercept is to set x=0. Y=0 is also known as zero. Once the equation is solved, the answer is zero. For example, if the equation is y = a(x + r1)(x + r2), the y-coordinate of the vertex is -9. Another way to find the y-intercept is by substituting x into the original equation. If the equation is y = -3×2 – 3x+1, the vertex is -3. Graphing the equation will allow you to confirm the answer.
Read Also: How To Use Sohcahtoa?
The y-intercept of a line can be found by using the same method. By dividing the y-coordinates by the x-coordinates, you can determine the slope. However, a quadratic equation has one or more x-intercepts, meaning that the graph passes through two or more points along the x-axis. These points can be identified by running, graphing, or by using a table.
In quadratic equations, there are three general forms. One of them is the standard form. The other two are the factored and vertex form. Each of these has its own advantages and disadvantages. To summarize, the standard form of the quadratic equation is the most common. Unlike the other two forms, the standard form requires that the x-value be zero. Thus, it is the best option to use in most situations.
Finding the y-intercept from a graph and table
Whether you are graphing a function or determining the y-intercept of a line, you’ll want to make sure you have a good grasp of the basics before you begin. By understanding the y-intercept, you’ll be able to easily determine the x-coordinates of a point on a graph. This will allow you to use that information to graph the function and find the y-intercept.
The y-intercept is a point where a straight line crosses the y-axis in a plane. In many cases, the point can be found with a simple equation. It is also a good idea to print out a worksheet before beginning. Once you’re comfortable, you can move on to more complicated problems.
Oftentimes, a simple equation can be used to find the y-intercept of a function. There are many different ways to do this, though. Most equations include a constant term. A few of these include y=f(x). If you can get a hold of this formula, you’ll know the y-intercept of a particular function. You can solve this equation algebraically or simply by dividing the x-coordinates.
If you’re unsure of how to find the y-intercept of your function, try the slope-intercept form of the equation. This form includes the y-intercept as a constant. This means you can use the equation as a basis for writing functions.