How to Find the Vertex of a Quadratic Function
Finding the vertex of a quadratic equation can be a tricky thing to do. First you need to know whether the equation you’re working with has a maximum or a minimum. You also need to line it up with the general standard form of the equation. There are a few ways to do this.
Finding the vertex in a quadratic equation
A quadratic equation is an equation that can be solved for x and y. A quadratic equation can be written in general or standard form. The difference between the two forms is that the y coordinate in the standard form is 0 and in the vertex form it is -1.
Generally, the vertex is the point on the graph that defines the domain of the quadratic function. It is the lowest or highest point of the quadratic. Using calculus, it is possible to determine the vertex. To do this, you first need to find the x-coordinate and the derivative. You can then plug these values into a quadratic formula to get the y value.
Finding the vertex in a quadratic equation is often complicated and requires a great deal of knowledge of quadratic functions. Once you have determined the x-coordinate and the derivative, you can calculate the vertex. Depending on the type of quadratic you are solving, there are several ways to do this.
Probably the easiest way is to use the vertex form of a quadratic function. This form is a special form of the function that shows the minimum and maximum points on the graph. As a result, the vertex form is very easy to convert to the standard form.
Finding the vertex by lining it up with the general standard form
The vertex of a quadratic equation is the point that is the minimum and maximum of the function. In general, the lowest value will occur when the base of the quadratic term is zero. Depending on the function, it may have no x-intercepts or x-intercepts.
Students can use equations to find the vertex of a parabola. These equations are written in the standard form of the function. This form will allow students to find y-intercepts and leading coefficients. By graphing the quadratic function, students will be able to observe the increasing and decreasing portions of the parabola. They will also be able to see how the parameters affect the graph.
Read Also: How to Find the Perimeter of a Triangle
Finding the vertex of a quadratic function requires students to find y-coordinates and x-coordinates. When the y-coordinate is substituted into the equation, students will be able to determine the vertex.
The standard form is similar to the vertex form. However, the letters tell more information about the parabola. For example, the “a” value is used to determine the direction of the opening of the parabola. Increasing the “a” value results in a more narrow parabola. Increasing the value of the “a” never equals a value of 0.
The axis of symmetry is the vertical line that passes through the vertex. Axis of symmetry divides the parabola into two mirrored halves. This mirrored image is not part of the graph.
Students can also find the axis of symmetry by using a formula. The formula will add two intercepts and subtract the h value. Once the axis of symmetry is found, the student can find the vertex.
Finding the vertex by identifying (h, k)
If you are trying to learn how to find the vertex of a quadratic function, then you have come to the right place. Here you will find a few tips to help you.
The first thing to do is to find out which type of equation is best suited for your situation. You might be working on a linear equation, or you may be dealing with a parabola. This will help you narrow down the equation and identify the x-coordinates of the vertex.
You should also be sure to check the vertex on the graph of the equation to make sure it is on the correct side. You can either use the vertex form calculator, or you can simply do the math yourself.
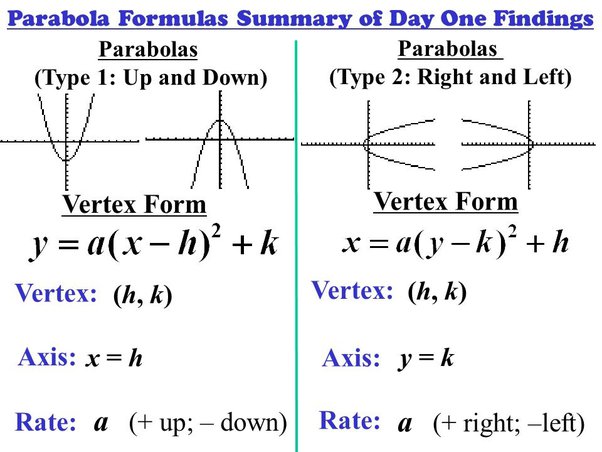
The vertex is also the lowest point in the equation. To identify this point, you will need to calculate a few nifty physics formulas. For example, if you are looking to find the y-coordinate of the vertex, then the answer is y = a(x – h)2 + k.
One of the most important things to remember is that a parabola’s vertex is not always at 0 – if it is, then the equation is useless. In this case, the vertex is located at a certain distance from the origin.
In order to understand which one is the best, you should also check out the other important mathematical properties of a parabola. These include the zero product property, the binomial equation, and the vertex form. When you are done with your homework, try to draw a parabola.
Finding the vertex by determining whether you have a minimum or maximum
Vertex is the point in a quadratic function that is the minimum or maximum value of that function. When we write a quadratic function in standard form, we can often find the vertex. This is because the range of a quadratic function is usually defined by the vertex. However, the vertex of a quadratic function can vary depending on the function itself.
The standard form for a parabola is y = ax2 + bx + c. The vertex of the parabola is the y-coordinate of the maximum or minimum point on the graph. If a parabola has an a>0, it will open upwards; if it has an a0, it will open downwards.
Read Also: Learning More About Percent Change Formula
A quadratic function is also written in general form. This means that the vertex can be found even if the function has no x-intercept. It can also be found in the case of a quadratic function with only one x-intercept. Similarly, the vertex of a quadratic functions with two x-intercepts is the y-coordinate of the minimum point on the graph.
Finding the vertex of a function is important for real world problems. For example, a company might want to know the point on a graph where the total profit or cost of a product is the lowest. In other instances, the vertex is the point on a graph where a horizontal parabola crosses the x-axis.