How to Find the Area of a Trapezoid
When you have a trapezoid that is the same length as its parallel sides, how can you determine the area? The answer is to use the Pythagoras’ theorem. If you know the length of the other two sides, you can calculate the area using the formula, A = h.
Pythagoras’ theorem
If you have ever wondered what the area of a trapezoid is, there are several ways to get the answer. Some people choose to use Pythagoras’ theorem to find the area of a trapezoid. This is a simple formula that is based on a trapezoid’s height and diagonals. It can also be used to calculate the area of an irregular trapezoid.
The area of a trapezoid, also known as the trapezoid’s perimeter, is the square-footage of the square that is enclosed by four sides. Depending on the base size, the total area can range from seventy-five to 108 square feet.
When you are using the Pythagorean theorem to find the area of the trapezoid, you will be able to see the relationship between the angles of the diagonals. A right triangle has two bases, one of which is called the hypotenuse. The other base is called the apex. In the Pythagorean theorem, the length of a base equals the length of the other base.
Read Also: sohcahtoa
To calculate the area of a trapezoid, you need to determine the angle between the diagonals and the height of the triangle. You can do this by drawing a line from the midpoint of the two sides. Then, add the areas of each side and the diagonals to determine the total area of the triangle.
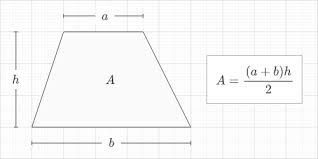
Area = 1/2 (a+b)h
The area of a trapezoid is an arithmetic quantity that can be easily calculated. It is the ratio of a pair of parallel sides to the perpendicular distance between them. This is often called the Pythagorean theorem.
In general, a trapezoid has a pair of parallel sides, a pair of lateral or non-parallel sides, and a height. However, not all of the three are necessarily the same. So, you may have to break your trapezoid into a few simpler shapes.
Read Also: difference quotient calculator
A trapezoid with a base length of 9 cm and a height of 3 cm has an area of 21 square centimeters. Similarly, a trapezoid with a base height of 8 cm and a base length of 13 cm has an area of 73.5 centimeters.
The area of a trapezoid can be calculated with the help of a calculator or by using a formula. As mentioned before, the formula can be found by dividing the height of a trapezoid into its parallel sides. Another simple method is to multiply the sum of its parallel sides by the height of the trapezoid.
Calculating the area of a trapezoid involves the aforementioned Pythagorean theorem and a similar process used by ancient Egyptians. However, it is important to note that this calculation is not as easy as it may seem.
This is because, in addition to being a quadrilateral, the trapezoid has two pairs of bases. One of the bases is the top or bottom, while the other is the side. To find the height of a trapezoid, you must first determine the perpendicular distance between the top and bottom of each base.
Isosceles trapezoid
An isosceles trapezoid is a quadrilateral whose non-parallel sides and bases are of equal length and are congruent. The area of this quadrilateral is a function of the height and diagonals of the trapezium.
To calculate the area of an isosceles trapezoid, you can use the Pythagorean theorem. In this method, you first have to solve for the height and the length of the angled sides. Once you have those values, you can then calculate the area.
Isosceles trapezoids are special types of trapezoids, which are characterized by having a line of symmetry joining the midpoint of two parallel sides. This line of symmetry is also referred to as the axis of symmetry. If there is only one axis of symmetry, the trapezoid is called a kite. Alternatively, if there are two axes of symmetry, the trapezoid can be referred to as a rhombus.
Typically, the diagonals of an isosceles trapezoid are of equal length, and divide into segments of equal length, as shown in the diagram above. When these diagonals cross each other at the center, they have a perpendicular bisector. This perpendicular bisector is the symmetry axis.
An isosceles trapezoid can be divided into four basic parts: the base, the legs, the diagonals, and the height. You can calculate the height of the trapezoid by subtracting the height of the diagonals from the height of the bases.
Finding the area of a trapezoid with parallel side lengths
When it comes to finding the area of a trapezoid with parallel side lengths, there are several different methods you can use. Using the Pythagorean theorem, you can find the perimeter of the trapezoid by adding the four sides. But you can also calculate the area of the trapezoid if you know the height and the length of the diagonals.
A trapezoid is a geometric shape composed of two triangles, a and b, and one rectangle. The parallel sides of the rectangle are the sides of the trapezoid. You can also calculate the area of a trapezoid using the area formula for a parallelogram, which is Area = (a+b)/2 h. This is a simple calculation that requires you to calculate the length of the base, the length of the nonparallel side, and the height.