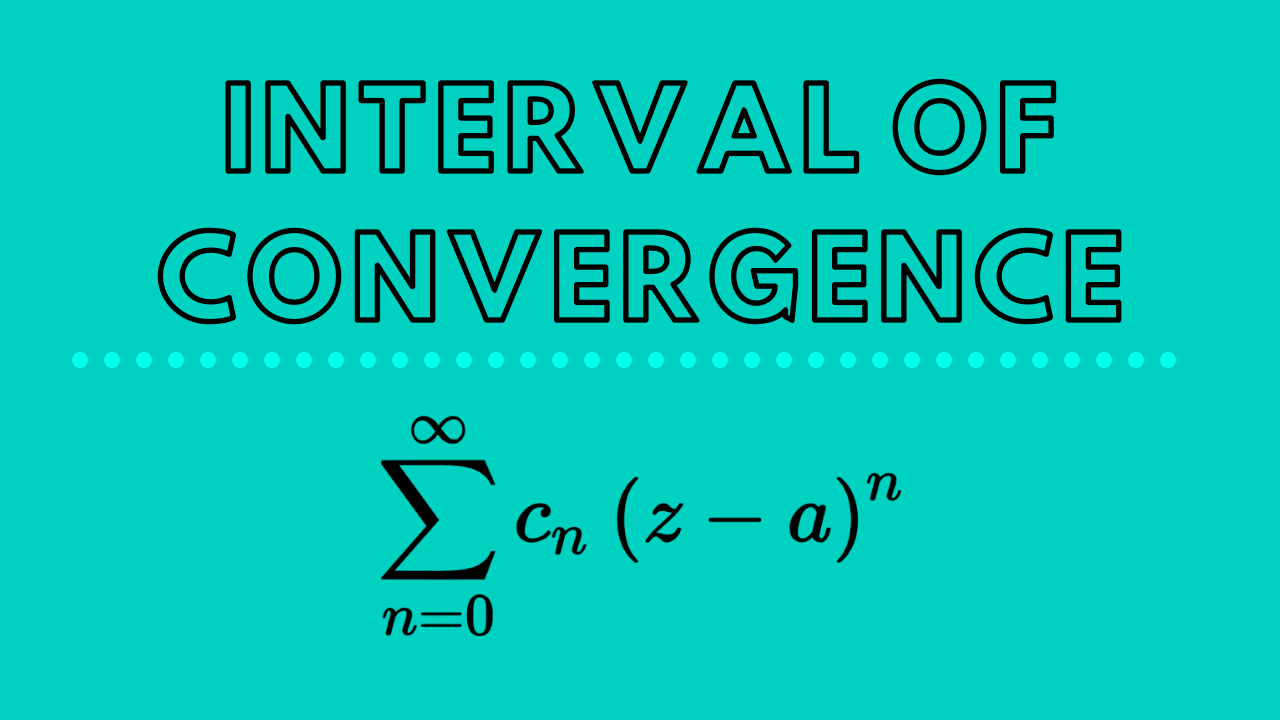
About interval of convergence calculator
The interval of Convergence Calculator is a cost-free device that shows the convergence point for any offered series. The online radius of convergence calculator makes calculations much faster, and also it shows the convergence point in a split second.
How to use the interval of convergence calculator?
To utilize the radius of convergence calculator, adhere to these actions:
1: Enter the feature as well as range in the proper input fields
2: Currently click “Determine” to calculate the outcomes
3: In the brand-new window, you will undoubtedly locate the convergence point for the given series
What is the Radius of Convergence or interval of convergence calculator?
When a first-year university student, you are instructed on boundless the radius and series of convergence. The series is a polynomial in a variable X with an unlimited number of terms.
With a bit of initiative, you can encourage on your own that the unlimited amount does not make sense if X is more significant than one or smaller than minus one (it diverges). Can provide the amount for X smaller sized than one and more meaningful than minus one. This feature has a radius of convergence of one.
We need to point out four more things.
Mathematicians call it a radius of convergence since they like to connect in complex numbers, like X, = U + i V, where i * i = -1. They can reveal that the series converges as the radius of convergence increases U ² + V ²= R ² and deviates outside the circle.
You can deduce that our amount (with all Ki= 1) will constantly give 1/( 1-X) when it merges. The series represents a feature in this feeling. Taylor’s thesis clarifies precisely how to get the series from the function. Can likewise use various other infinite series Ki to make other features.
FAQs on interval of convergence calculator
Exactly how do you discover the radius of convergence?
The radius of convergence is half the size of the convergence interval. If the radius of convergence is R, the period of convergence consists of the open period: (a − R, a + R).
No matter the option of x, the series merges with the Proportion Examination. As a result, the radius of convergence is R= ∞, and the period of convergence is (− ∞, ∞).
Why is it referred to as a radius of convergence?
It’s called a radius of convergence since mathematicians like to plug in complex numbers, like X = U + i V, where i * i = -1. Using this series, they can show that
2+2=2 assembles inside the circle yet diverges outside it.
Can the radius of convergence be 0?
The distance between the centre of a power series’ convergence interval and its endpoints. The radius of convergence is zero if the series only merges at a single point.
What is the radius of convergence of a power series?
In maths, the radius of convergence of a power series is the radius of the enormous disk in which the series merges.
Precisely how do you examine for convergence?
When the limit of a [n]/ b [n] is positive, after that, the amount of a [n] merges if and also just if the sum of b [n] converges.
However, when the limit of a [n]/ b [n] is no, and also the sum of b [n] converges, after that, the sum of a [n] likewise merges.
Also, if the limit of a [n]/ b [n] is boundless, and the amount of b [n] splits, after that, the sum of an [n] additionally splits.
What is meant by the term convergence?
A procedure of convergence, especially the motion towards a point of union; the convergence of three rivers, mainly: worked with activity in between both eyes allowing a single point photo to be based on their retinas. Convergence is the state or residential or commercial property of being convergent.
What is the radius of convergence of a geometric series?
Suppose a power series merges only for x = a. In that case, the radius of convergence is defined as R = 0.– If the power series assembles for all values of x, then the radius of convergence is specified to be R = ∞.
What is the radius of convergence of the Maclaurin series?
The radius of convergence for a Maclaurin series can be discovered by checking which of the three situations we’re in. If our Maclaurin series assembles all real values, we state that our radius of convergence amounts to ∞.





